QF Boxing Day Competition
Get free access to Lie Groups with Applications.
Dear QF Community,
I hope you had a wondeful Christmas!
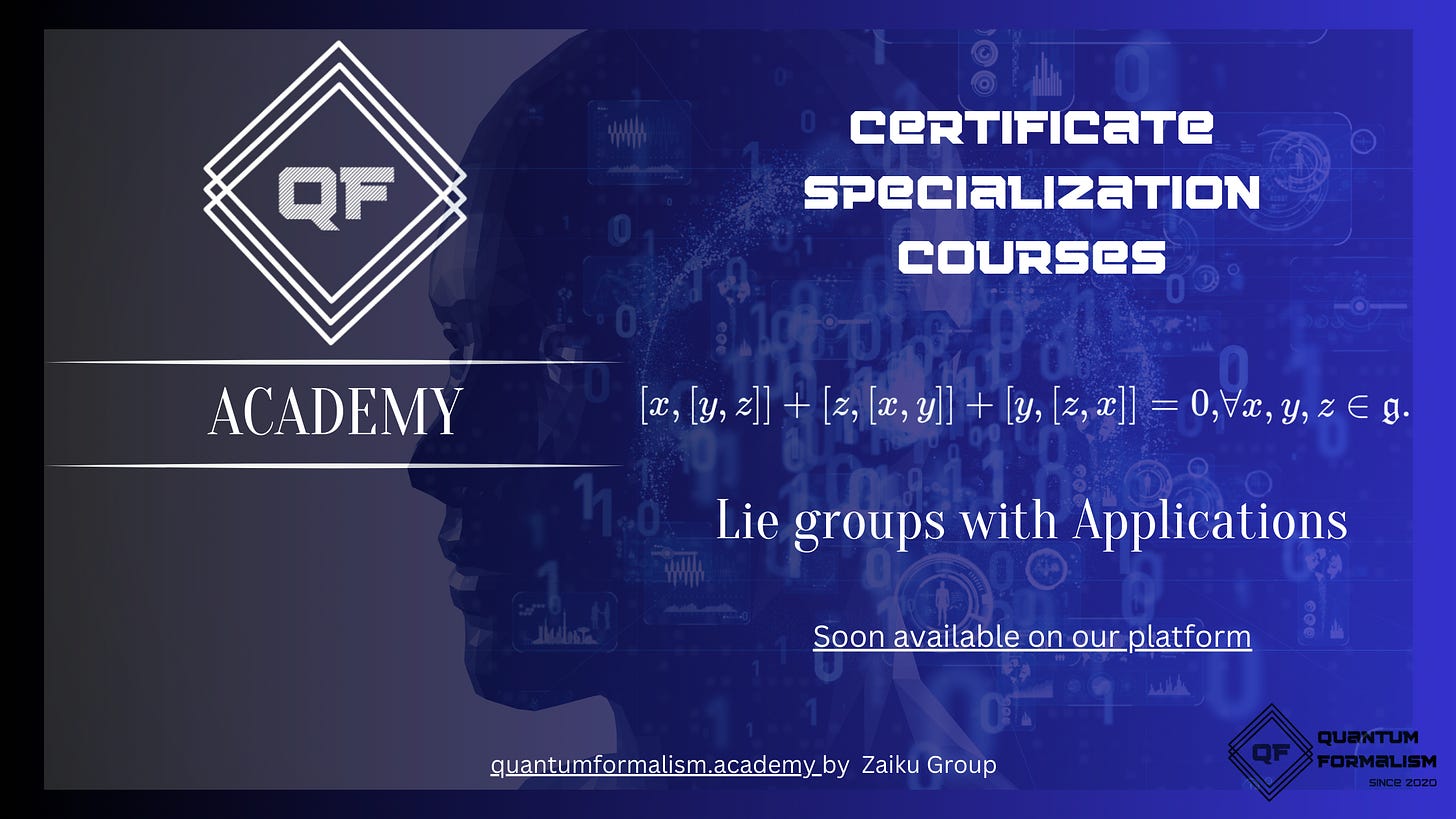
I am offering 15 free places to access Quantum Formalism's upcoming specialisation course on Lee Groups with Applications to Quantum Computing and Machine Learning.
To participate:
1) Follow the Quantum Formalism page (ignore if you're already following)
2) Like this post and comment something mathematically hilarious (e.g a joke)
3) Reshare the post with your thoughts about the importance of Lie Groups and why you would like to master the topic
Alternatively, you can still get access to the early bird Christmas deal via https://quantumformalism.academy/lie-groups-with-applications. Early bird sign ups will access our pre-course preparation help covering the following prerequisites:
Linear Algebra:
Vector spaces: Basis, dimension, and linear transformations.
Matrix properties: Determinants, eigenvalues, eigenvectors, and diagonalization. Jordan decomposition would be great.
Inner product spaces: Orthonormal bases and the Gram-Schmidt process.
Special matrices: Orthogonal, unitary, and Hermitian matrices.
Group theory:
Basic definitions: Groups, subgroups, cosets, and normal subgroups. I was planning on reviewing some of this in the first lecture, but only for a few minutes or so!
Homomorphisms: Definitions, kernels, and images.
Actions: Group actions, orbits, stabilizers, and applications to symmetry.
Topology and Smooth Manifolds:
Basic topology: Open and closed sets, continuous maps, homeomorphisms, connectedness and compactness.
Differentiable structures: Charts, atlases, and smooth maps.
Tangent spaces: Definition, derivations, and examples.
Submanifolds: Embedded submanifolds and the implicit function theorem.
I will announce the winners in the new year before the course is available.
Good luck! ;)
Have a great week ahead!
Best wishes,
Bambordé
Quantum Formalism (QF) Academy Team
